C04 MODERN PORTFOLIO THEORY AND CAPM

EXPECTED RETURN & VOLATILITY OF A TWO ASSET PORTFOLIO #

The expected return on a portfolio is a weighted average of the expected returns on the individual assets that are included in the portfolio. For a two-asset portfolio:

E(R_p) = w₁E(R₁) + w₂E(R₂)

where: E(R_p) = expected return on Portfolio P

w_i = weight of the portfolio allocated to Asset i

E(R_i) = expected return on Asset i

The weights (w1 and w2) must sum to 100% for a two-asset portfolio.

The variance of a two-asset portfolio equals:

σ²_p  = w²₁ σ₁²  + w²₂ σ²₂ + 2w₁w₂ σ₁σ₂ρ_1,2

Where ρ = correlation coefficient between asset 1 & asset 2.

THE PORTFOLIO POSSIBILITIES CURVE #

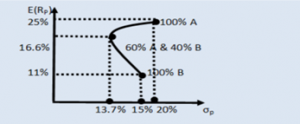
• If 100% of the portfolio is allocated to A, the portfolio will have the expected return and standard deviation of A (i.e., A is the portfolio), and the investment return and risk combination is at the lower end of the curve.
• As the investment in A is decreased and the investment in B is increased, the investment moves up the curve to the point where the portfolio’s expected return is 16.6% with a standard deviation of 13.72% (labelled 60% A 40% B).
• Finally, if 100% of the portfolio is allocated to B, the portfolio will have the expected return and standard deviation of B, and the investment return and risk combination is at the upper end of the curve.

THE MINIMUM VARIANCE PORTFOLIO #

The minimum variance portfolio is the portfolio with the smallest variance among all possible portfolios on a portfolio possibilities curve.  On the portfolio possibilities curve, the minimum variance portfolio represents the left-most point on the curve.

THE SHAPE OF THE PORTFOLIO POSSIBILITY CURVE #

The shape of the portfolio possibilities curve is best described in two pieces.

The piece of the portfolio possibilities curve that lies above the minimum variance portfolio  is concave.

The piece of the portfolio possibilities curve that lies below the minimum variance portfolio is convex.

CORRELATION & PORTFOLIO DIVERSIFICATION #

PERFECT POSITIVE CORRELATION

In the case where two assets have perfect positive correlation (i.e., p = 1), the portfolio standard deviation reduces to the simple weighted average of the individual standard deviations indicating no diversification.

σ_p = w₁σ₁ + w₂σ₂

PERFECT NEGATIVE CORRELATION

The greatest diversification is achieved in the case where two assets have perfect negative correlation (i.e., p = —1). In this case, the portfolio standard deviation reduces to two linear equations

σ_p = w₁σ₁ – w₂σ₂  OR  σ_p = -w₁σ₁ + w₂σ₂

ZERO CORRELATION

When the correlation between two assets is zero, the covariance term in the portfolio standard deviation expression is eliminated, and the resulting expression is:

σ_p = [w²₁σ²₁ + w²₂σ²₂]^1/2

MODERATE POSITIVE CORRELATION

Two assets are moderately correlated (e.g., p = 0.5), then the portfolio standard deviation reduces to:

σ_p = [w²₁σ²₁ + w²₂σ²₂ + w₁w₂σ₁σ₂]^1/2

THE EFFICIENT FRONTIER #

Plotting all risky assets and potential combinations of risky assets will result in the following graph.

Portfolios such as D and E are called efficient portfolios, which are portfolios that have:

• Minimum risk of all portfolios with the same expected return.
• Maximum expected return for all portfolios with the same risk.

The efficient frontier is a plot of the expected return and risk combinations of all efficient portfolios, all of which lie along the upper-left portion of the possible portfolios .

SHORT SALES & THE EFFICIENT FRONTIER

When allowing for short sales, the efficient frontier expands up and to the right. By shorting, it is possible to create higher return and higher volatility portfolio combinations that would not be possible otherwise.

THE CAPITAL ASSET PRICING MODEL #

The CAPM provides a way to calculate an asset’s expected return (or “required” return) based on its level of systematic (or market-related) risk, as measured by the asset’s beta.

CAPM ASSUMPTIONS:

• Markets are frictionless i.e. no taxes or transaction cost.
• Assets are infinitely divisible
• Market is perfectly competitive.
• Investors have homogeneous expectations.
• Investors can borrow and lend unlimited amounts at the risk-free rate. Investors have same holding period. Unlimited short selling is allowed. All assets are marketable.

THE CAPITAL MARKET LINE #

In the presence of riskless lending and borrowing, the efficient frontier transforms from a curve to a line tangent to the previous curve. Investors will choose to invest in some combination of their tangency portfolio and the risk-free asset. Assuming investors have identical expectations regarding expected returns, standard deviations, and correlations of all assets, there will be only one tangency line, which is referred to as the Capital market line (CML). The equation for the CML is:

E(R_P) = R_F + (E(R_M ) – R_F )/σ_M)  σ_P

The slope of the CML is often called the market price of risk and equals the reward-to-risk ratio (or Sharpe ratio) for the market portfolio. This is calculated as:

(E(R_M) – R_F)/ σ_M

DERIVING THE CAPM #

There are three major steps in deriving the CAPM:

1. Recognize that since investors are only compensated for bearing systematic risk, beta is the appropriate measure of risk.
2. By knowing that portfolio expected return is a weighted average of individual expected returns and portfolio beta is a weighted average of the individual betas, we can show that portfolio return is a linear function of portfolio beta. Since arbitrage prevents mispricing of assets relative to systematic risk (beta), an individual asset’s expected return is a linear function of its beta.
3. Use the risk-free asset and the market portfolio, which  are two points on the security market line, to solve for  the intercept and slope of the CAPM. The equation for CAPM is: E(R_i)=R_F + [E(R_M) – R_F] βi

BETA #

The sensitivity of an asset’s return to the market return is referred to as the asset’s beta. Beta is a standardized measure of the covariance of the asset’s return with the market return. Beta can be calculated as follows:

β_i = Cov_i,M / σ²_M

The least squares regression line is the line that minimizes the sum of the squared distances of the points plotted from the line (this is what is meant by the line of best fit). The slope of this line is our estimate of beta.

PORTFOLIO BETA

The beta of a portfolio is the sum of the weighted individual asset betas within a portfolio.

MEASURES OF PERFORMANCE #

Modern portfolio theory and the CAPM are built upon the link between risk and return. Three measures exist to assess an asset’s or portfolio’s return with respect to its risk.

The Treynor measure is equal to the risk premium divided by beta, or systematic risk:

Treynor measure of a portfolio =  (E(RP) – RF)/βP

The Sharpe measure is equal to the risk premium divided by the standard deviation, or total risk:

Sharpe measure of a portfolio =  (E(RP)-RF)/σP

The Jensen measure (or Jensen’s alpha or just alpha), is the asset’s excess return over the return predicted by          the CAPM:

Jensen measure of a portfolio = E(Rp) – [RF +[E(RM) – RF]βP]

The three risk measures above give different perspectives and may give different rankings for portfolios. A portfolio with low diversification may have a higher Treynor measure, a higher alpha, but a lower Sharpe measure than another portfolio.

Alpha can be modified by the use of other reference portfolios.

TRACKING ERROR, INFORMATION RATIO & SORTINO RATIO #

Tracking error

Tracking error is the term used to describe the standard deviation of the difference between the portfolio return and the benchmark return. Typically, the manager must keep the tracking error below a stated threshold.

Tracking Error = σ_ep

Information Ratio

The information ratio is essentially the alpha of the managed portfolio relative to its benchmark divided by the tracking error.

Information Ratio = E(R_P ) – E(R_B ) /σep

Sortino ratio

The measure of risk in the Sortino ratio is the square root of the mean squared deviation from RMIN  of those observations in time periods t where RPt < RMIN , else zero. Letting RMIN denote the minimal acceptable return and MSDMIN the risk measure:

Sortino Ratio = E_(RP) – R_Min /Root( MSD)_MIN

Where:

MSD_MIN  =  Ʃ (R_Pt—R_MIN)²/N

The Sortino ratio can be interpreted as a variation of the Sharpe ratio that is more appropriate for a case where returns are not symmetric.